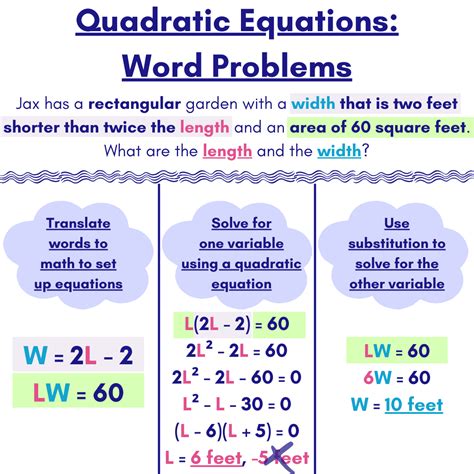
Quadratic equations are a fundamental concept in mathematics, and solving them can be a challenge, especially when it comes to word problems. However, with the right approach, you can solve quadratic word problems with ease. In this article, we will explore the steps to solve quadratic word problems in a simple and effective way.
Understanding Quadratic Word Problems
Quadratic word problems involve solving equations that can be written in the form ax^2 + bx + c = 0, where a, b, and c are constants. These problems often involve real-world scenarios, such as projectile motion, optimization problems, and geometry. The key to solving quadratic word problems is to identify the variables, constants, and the relationship between them.

Step 1: Read and Understand the Problem
The first step to solving a quadratic word problem is to read and understand the problem carefully. Read the problem multiple times until you are sure you understand what is being asked. Identify the variables, constants, and the relationship between them. Ask yourself questions like:
- What is the problem asking me to find?
- What are the variables and constants involved?
- What is the relationship between the variables and constants?
Example Problem
A ball is thrown upwards from the ground with an initial velocity of 20 m/s. The height of the ball above the ground is given by the equation h(t) = -5t^2 + 20t, where h(t) is the height in meters and t is the time in seconds. Find the time it takes for the ball to reach its maximum height.
Step 2: Write the Equation
Once you have identified the variables, constants, and the relationship between them, write the equation that represents the problem. Use the information given in the problem to write the equation. Make sure to include all the variables and constants in the equation.
Example Equation
In the example problem, the equation is already given as h(t) = -5t^2 + 20t. However, if the equation was not given, you would need to use the information provided to write the equation.
Step 3: Solve the Equation
Now that you have written the equation, solve it to find the value of the variable. You can use various methods to solve the equation, such as factoring, quadratic formula, or graphing.
Example Solution
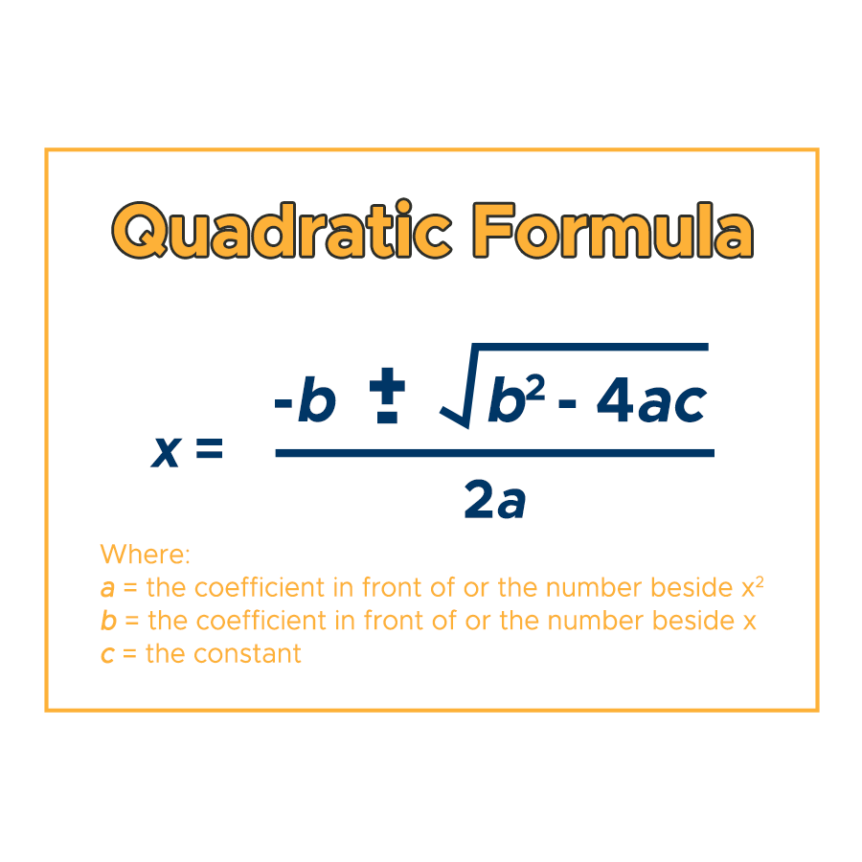
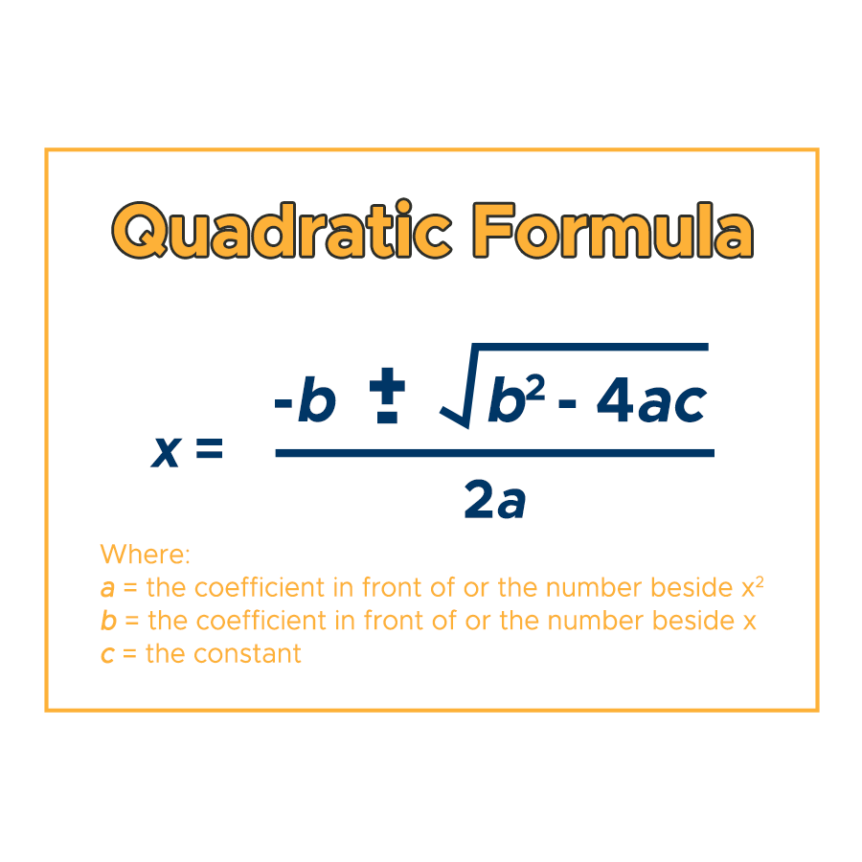
In the example problem, we need to find the time it takes for the ball to reach its maximum height. To do this, we can use the quadratic formula:
t = (-b ± √(b^2 - 4ac)) / 2a
where a = -5, b = 20, and c = 0.
Plugging in the values, we get:
t = (-20 ± √(20^2 - 4(-5)(0))) / 2(-5) t = (-20 ± √(400)) / (-10) t = (-20 ± 20) / (-10) t = 0 or t = 4
Since time cannot be negative, the only valid solution is t = 4 seconds.
Step 4: Check the Solution
Finally, check the solution to make sure it makes sense in the context of the problem. Plug the solution back into the original equation to ensure that it satisfies the equation.
Example Check
In the example problem, we found that t = 4 seconds. Plugging this value back into the original equation, we get:
h(4) = -5(4)^2 + 20(4) h(4) = -5(16) + 80 h(4) = -80 + 80 h(4) = 0
Since the height of the ball is 0 at t = 4 seconds, our solution is valid.

Gallery of Quadratic Word Problems
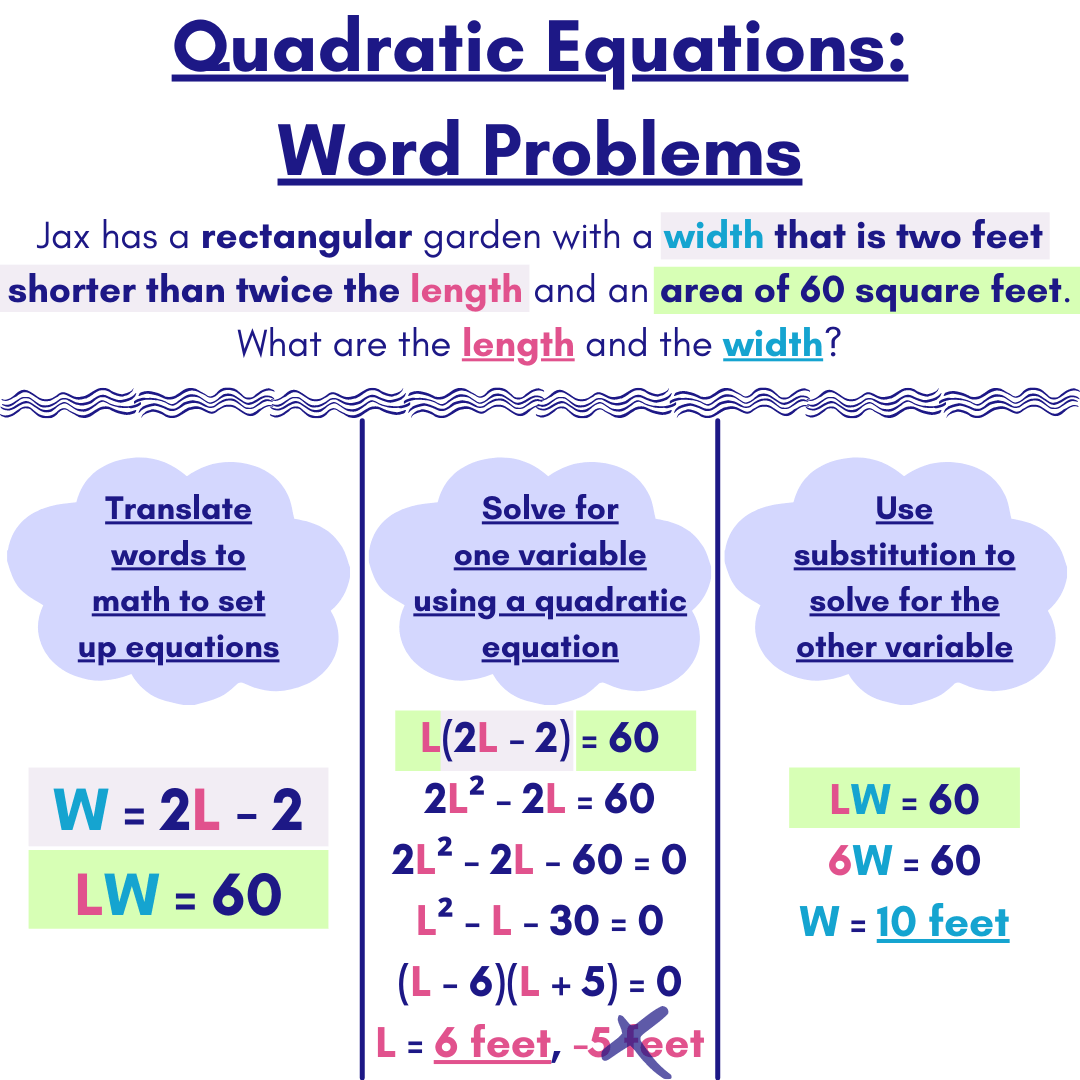
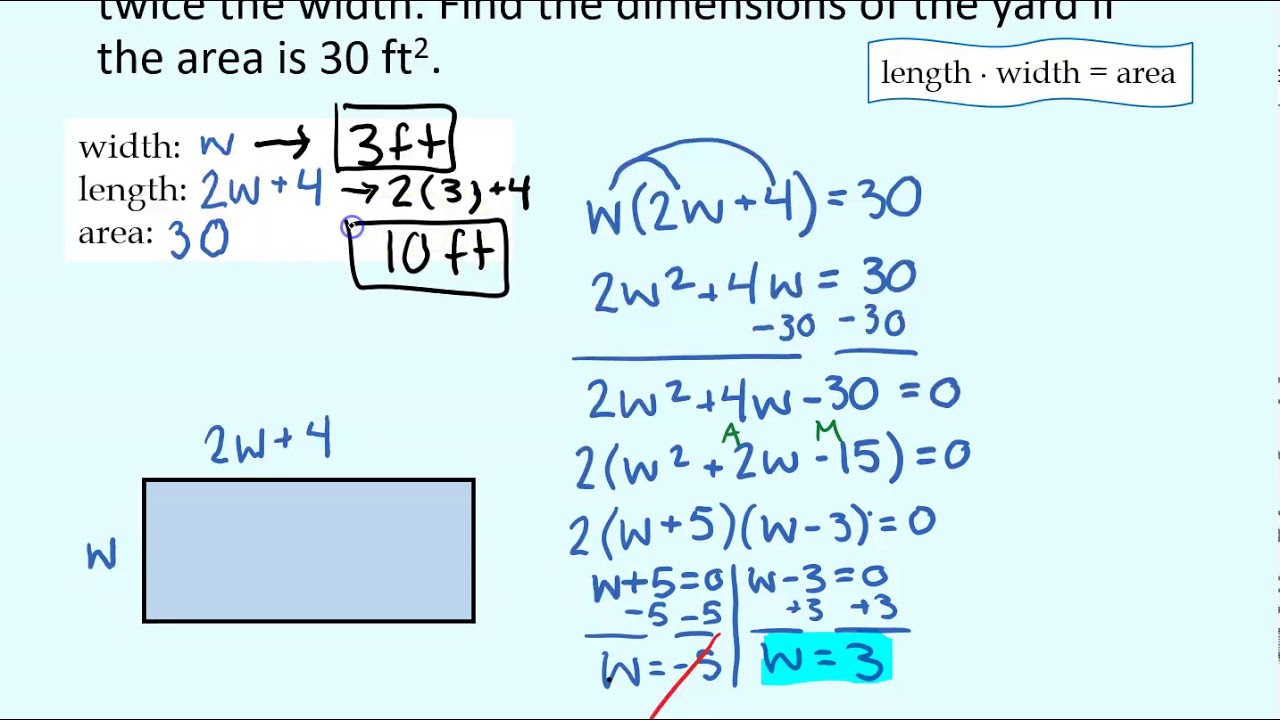
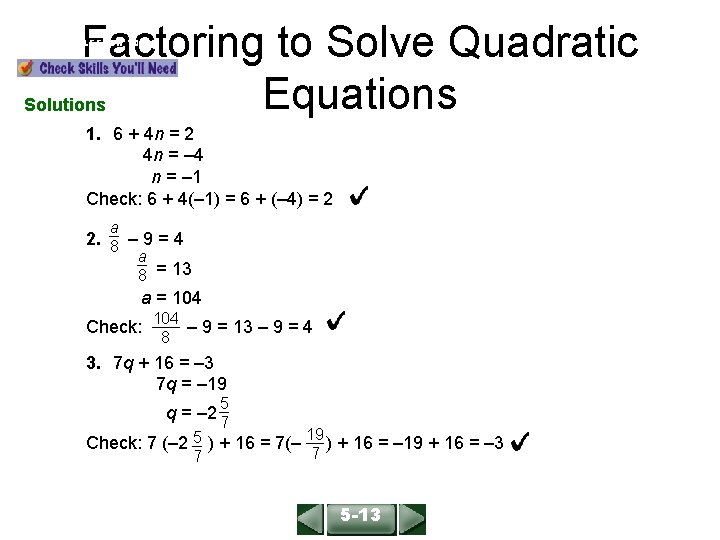
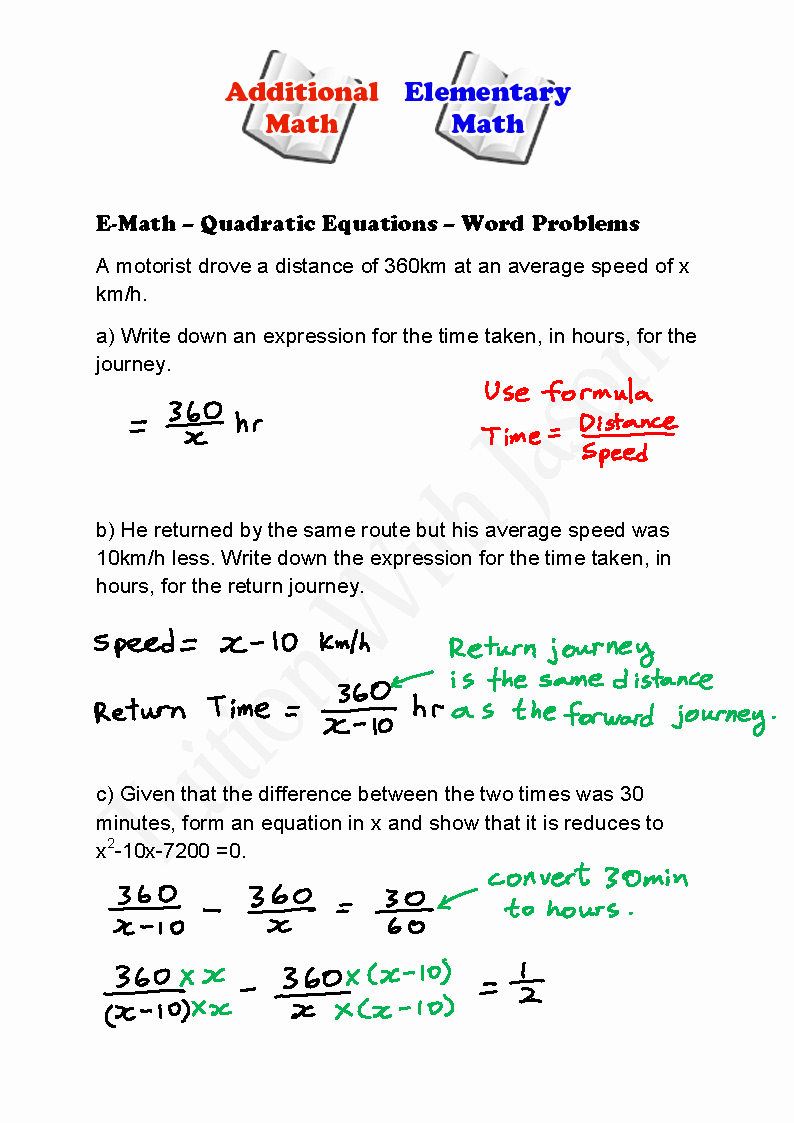
What is a quadratic word problem?
+A quadratic word problem is a problem that involves solving a quadratic equation to find the solution.
How do I solve a quadratic word problem?
+To solve a quadratic word problem, follow the steps outlined in this article: read and understand the problem, write the equation, solve the equation, and check the solution.
What is the quadratic formula?
+The quadratic formula is x = (-b ± √(b^2 - 4ac)) / 2a, where a, b, and c are the coefficients of the quadratic equation.
In conclusion, solving quadratic word problems can be challenging, but by following the steps outlined in this article, you can make it easier. Remember to read and understand the problem, write the equation, solve the equation, and check the solution. With practice and patience, you can become proficient in solving quadratic word problems.